Dimensionless numbers : a basic tool for the CFD engineer

Reynolds, Mach, Prandtl numbers… In fluid mechanics, it is very common to come across some of them, it is even probably impossible not to. Used wisely, they represent a real asset for modeling a problem, especially in the context of CFD. In this article, I offer a humble overview of these dimensionless numbers and their usefulness, for those unfamiliar with them.

Dimensionless numbers : what origin, what use?

A simple explanation

In physics, each quantity is expressed in units. For example, time can be expressed in seconds, hours, days. If the number of units is unlimited, these are on the other hand based on a finite size dimensional system: there are currently only 7 dimensions to describe all of current physics based on the international system: Mass , length, time, temperature, electric intensity, luminous intensity and quantity of matter.

As their name suggests, dimensionless numbers come from the concept of dimensional analysis (which is not unique to fluid mechanics!). Briefly, it is a general methodology for obtaining dimensionless physical relationships, with the aim of:

Reduce the number of variables needed to describe a physical phenomenon.
Study the asymptotic solutions of a law by identifying the preponderant terms.
Establish a link between systems of different dimensions (principle of similarity).

Several ways of proceeding are possible, the best known being the use of the Vaschy-Buckingham π theorem. This theorem makes it possible both to specify the number of independent dimensionless variables that can be constructed from the fundamental dimensional quantities of the problem, while constructing them afterwards. In the end, the result of a dimensional analysis is therefore to obtain dimensionless groups equivalent to the dimensional system.

A typical application in fluid mechanics

Let us take the example of the Navier-Stokes equation in a classic case: that of an incompressible fluid, with constant viscosity. It can be written as follows:

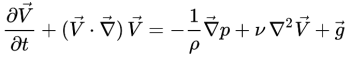
with V the velocity vector, p the pressure, ρ the density, ν the kinematic viscosity and g the force of gravity.

Without going into details, once the number of independent parameters has been determined, each physical quantity X can be broken down as the product of a dimensionless quantity X* by a reference dimension X0 linked to the problem, i.e. X = X0.X*

After a few manipulations, it is thus possible to obtain:

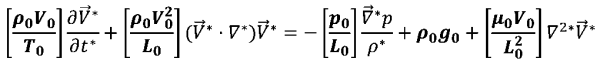
This equation can be made dimensionless by dividing it by one of the terms in square brackets. Quite often, regarding the Navier-Stokes equation, it is the term related to convective acceleration that is used for this. The equation then becomes:

This time, each term in square brackets is dimensionless. And it so happens that in this example, they represent four classical dimensionless numbers in fluid mechanics:

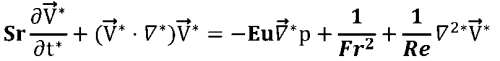
With :

Sr is the Strouhal number
Eu is the Euler number
Fr is the Froude number
Re is the Reynolds number

Note : Dimensionless numbers are usually named after the scientist who highlighted their interest.

A simplified phenomenological approach

Usefulness of dimensionless numbers is easily explained: they make it possible to quantify the relative importance of the various physical effects involved. Thus, if we start from the previous example:

The Strouhal number is formed from the ratio between the unsteady acceleration term and the convective acceleration term. It makes it possible to characterize the propensity of the flow to adopt or not an oscillatory movement.
The Euler number characterizes the relationship between the pressure term and the convective acceleration term. It makes it possible to evaluate the importance of the force of pressure compared to the force of inertia (in practice, one often finds an additional factor 2 with the denominator to introduce a concept of dynamic pressure).
The Froude number assesses the relationship between the inertia term and the gravity term. It is particularly important in the case of free surface flow, for example.
The Reynolds number characterizes the relationship between the inertia term and the viscosity term. This makes it possible to detect the nature of the flow (laminar or turbulent).

CFD application

As explained in a previous article, the first phase of a CFD engineer’s job is to correctly model the problem he is facing. This includes solving the right equations, taking the right terms into account, but also simplifying the problem when relevant. As such, the use of dimensionless numbers is of some interest:

This can help determine which models to use. For example, determining the characteristic Reynolds number of the problem makes it possible to know whether or not it is necessary to use a turbulence model.
This can also make it possible to neglect certain terms of the equations, in the case for example where the order of magnitude of one of the terms is clearly lower than that of all the others.

Classically, when a CFD engineer is confronted with a problem to be solved in fluid mechanics, he will therefore almost systematically seek to identify the dimensionless numbers characteristic of the problem in question, and to evaluate them.

Some frequently encountered dimensionless numbers

This selection is obviously anything but exhaustive, because in practice, there are a multitude of these numbers!

Note : a more complete list is for example available on Wikipedia.

Reynolds number

Denoted Re, it is defined as:

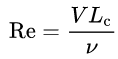
with V the characteristic velocity of the fluid, Lc the characteristic dimension of the flow and ν the kinematic viscosity of the fluid. It represents the ratio between the inertial forces and the viscous forces, and makes it possible to characterize the type of flow (laminar or turbulent).

Mach number

Denoted Ma, it is defined as :

where V is the velocity of the fluid and a is the speed of sound in the considered environment. It measures the relationship between the forces related to the movement and the compressibility of the fluid, and therefore makes it possible to evaluate the relevance of the hypothesis of incompressibility of the fluid, for example.

Prandtl number

Denoted Pr, it is defined as :

with ν the kinematic viscosity of the fluid, α its thermal diffusivity, μ its dynamic viscosity, λ its thermal conductivity and Cp its specific heat capacity. This number makes it possible to compare the rapidity of thermal phenomena and hydrodynamic phenomena in a fluid.

Froude number

Denoted Fr, it is defined as :

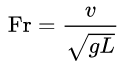
where v is the fluid velocity, g the gravity and L the characteristic length of the flow. As said in the previous paragraph, this number measures the ratio between the force of inertia and the force related to gravitation.

Rayleigh number

Denoted Ra, it is defined as :

with g the gravity, β the coefficient of volumetric thermal expansion of the fluid, α its thermal diffusivity, ν its kinematic viscosity, Ts the temperature of the wall, T∞ the temperature away from the wall, and Lc the characteristic dimension of the flow . This number makes it possible to characterize the heat transfer within a fluid, i.e. to evaluate the importance of convection compared to conduction. It can be noted that it can be defined from other dimensionless numbers, the Prandtl number Pr and the Grashof number Gr.

Conclusion

In practice, the existing dimensionless numbers are very numerous. Some appear in most fluid mechanics problems encountered, while others are much more specific. With experience, the CFD engineer comes to know the critical values associated with some of these numbers, values which generally make it possible to characterize a change in the nature of the flow.

If you liked this article, or if you want to discuss it, do not hesitate to show up!

See you soon!